Appoint description:
Description
A group of N people wishes to go across a river with only one boat, which can at most carry two persons. Therefore some sort of shuttle arrangement must be arranged in order to row the boat back and forth so that all people may cross. Each person has a different rowing speed; the speed of a couple is determined by the speed of the slower one. Your job is to determine a strategy that minimizes the time for these people to get across.
Input
The first line of the input contains a single integer T (1 <= T <= 20), the number of test cases. Then T cases follow. The first line of each case contains N, and the second line contains N integers giving the time for each people to cross the river. Each case is preceded by a blank line. There won't be more than 1000 people and nobody takes more than 100 seconds to cross.
Output
For each test case, print a line containing the total number of seconds required for all the N people to cross the river.
Sample Input
1 4 1 2 5 10
Sample Output
17
H:过河问题
#include<iostream>
#include<stdio.h>
#include<string.h>
#include<algorithm>
using namespace std;
int a[1005];
int main()
{
int t,n;
scanf("%d",&t);
while(t--)
{
scanf("%d",&n);
for(int i=0;i<n;i++)
scanf("%d",&a[i]);
sort(a,a+n);
if(n<=2)
{
printf("%d\n",a[n-1]);
continue;
}
int sum=a[1];//最后一定是 a[0].a[1]回去
while(n>3)
{
if(a[0]+a[n-1]+a[0]+a[n-2]>=a[0]+a[1]+a[n-1]+a[1])
sum+=a[0]+a[1]+a[n-1]+a[1];
else
sum+=a[0]+a[n-1]+a[0]+a[n-2];
n-=2;
}
if(n==3)
sum+=(a[0]+a[2]);
printf("%d\n",sum);
}
}
Appoint description:
Description
Assume the coasting is an infinite straight line. Land is in one side of coasting, sea in the other. Each small island is a point locating in the sea side. And any radar installation, locating on the coasting, can only cover d distance, so an island in the sea can be covered by a radius installation, if the distance between them is at most d.
We use Cartesian coordinate system, defining the coasting is the x-axis. The sea side is above x-axis, and the land side below. Given the position of each island in the sea, and given the distance of the coverage of the radar installation, your task is to write a program to find the minimal number of radar installations to cover all the islands. Note that the position of an island is represented by its x-y coordinates.
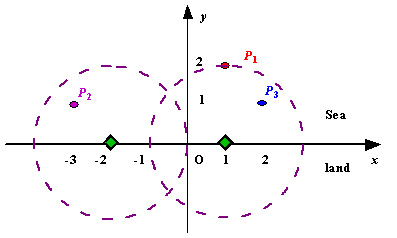
Figure A Sample Input of Radar Installations
We use Cartesian coordinate system, defining the coasting is the x-axis. The sea side is above x-axis, and the land side below. Given the position of each island in the sea, and given the distance of the coverage of the radar installation, your task is to write a program to find the minimal number of radar installations to cover all the islands. Note that the position of an island is represented by its x-y coordinates.

Figure A Sample Input of Radar Installations
Input
The input consists of several test cases. The first line of each case contains two integers n (1<=n<=1000) and d, where n is the number of islands in the sea and d is the distance of coverage of the radar installation. This is followed by n lines each containing two integers representing the coordinate of the position of each island. Then a blank line follows to separate the cases.
The input is terminated by a line containing pair of zeros
The input is terminated by a line containing pair of zeros
Output