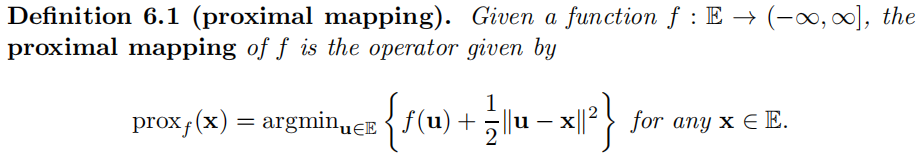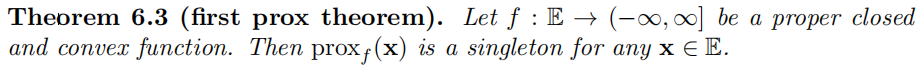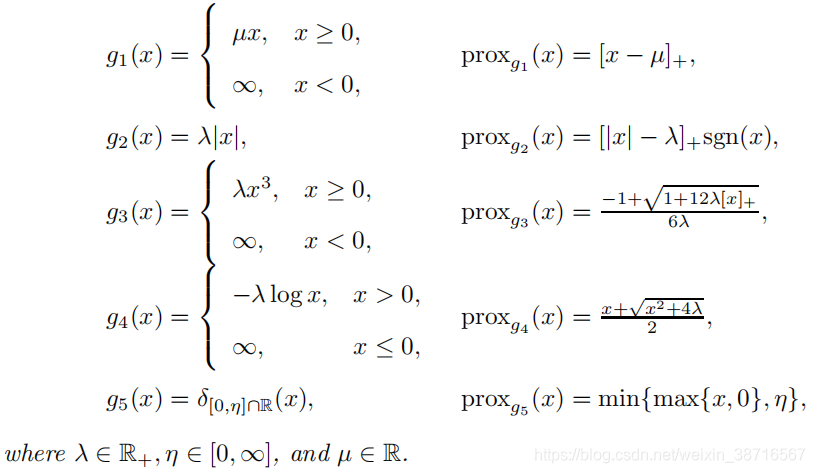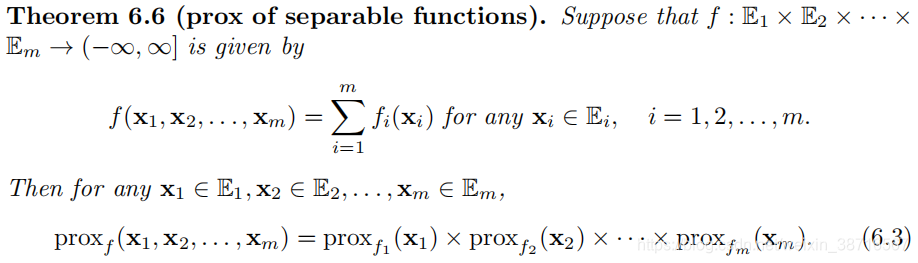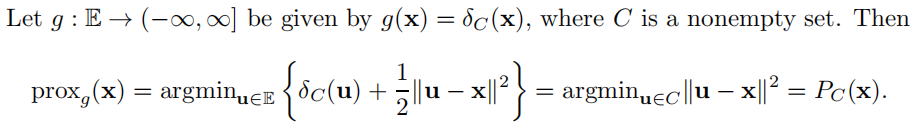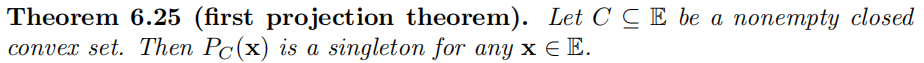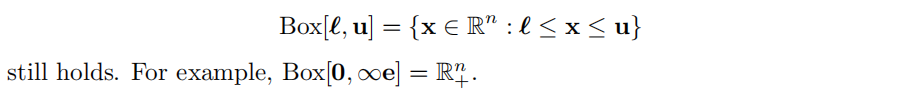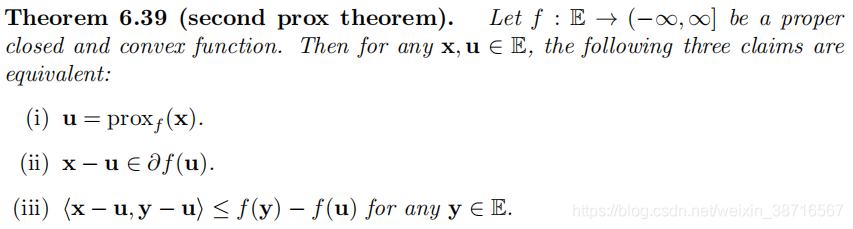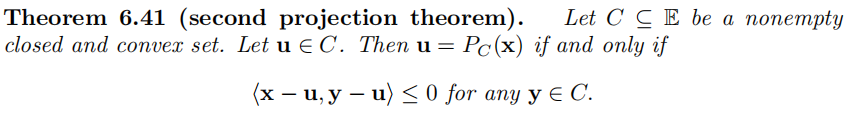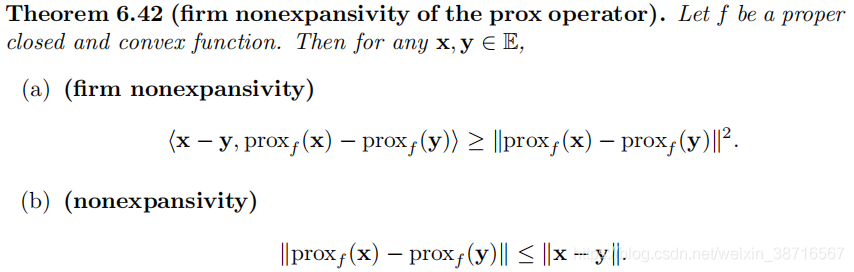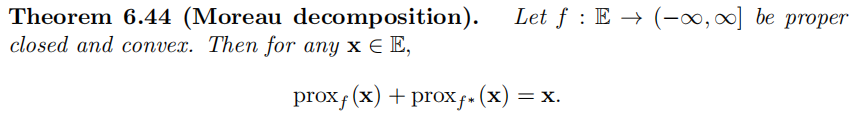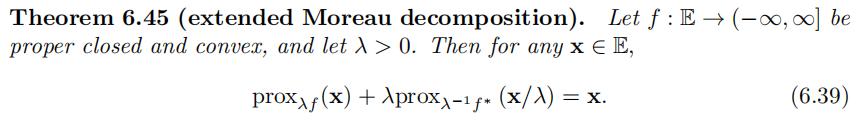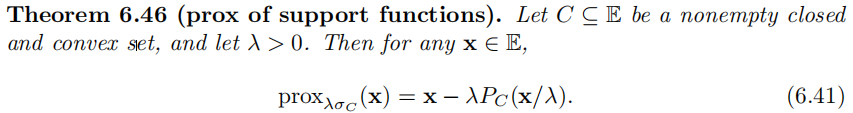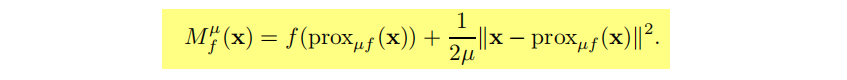本篇blog主要介绍了临近算子、临近算子与正交投影的联系、几个临近定理、Moreau分解等等
目录
1 Definition, Existence, and Uniqueness(什么是邻近算子、第一临近定理:临近算子解集只有一个元素 singleton、6.4 闭和coerciveness条件下邻近算子解集的非空性)
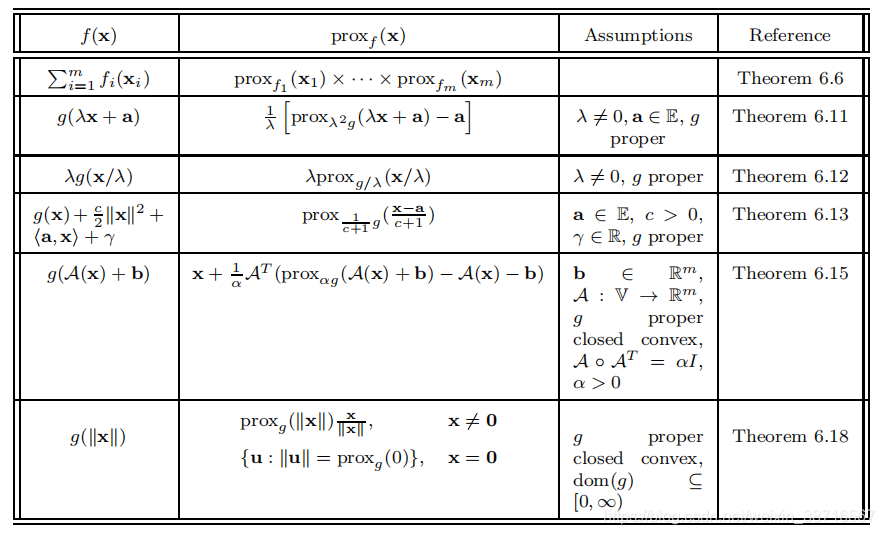
2 First Set of Examples of Proximal Mappings(临近算子的例子)(几种常见邻近算子的计算)
3 Prox Calculus Rules(临近算子的计算规则)(邻近算子的运算规则)
4 Prox of Indicators—Orthogonal Projections(指示函数的临近算子-正交投影)
4.1 The First Projection Theorem(也是singleton,其实可以引用第一临近定理证明,正交投影就是指数函数的临近)
4.3 Projection onto the Intersection of a Hyperplane and a Box
4.4 Projection onto Level Sets
5 The Second Prox Theorem(重点 3条等价性质 利用费马最优性条件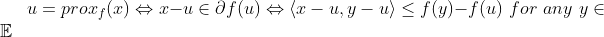
(第二投影定理与第二临近定理的第3条、邻近算子的非扩张性 联想到投影算子的非扩张性)
6 Moreau Decomposition(利用第二临近定理和共轭次梯度定理证明)(增广意义下的)
7 The Moreau Envelope(Moreau包络)
7.1 Definition and Basic Properties
7.2 Differentiability of the Moreau Envelope
7.3 Prox of the Moreau Envelope
8 Miscellaneous Prox Computations
1 Definition, Existence, and Uniqueness
f proper , closed , convex
2 First Set of Examples of Proximal Mappings(临近算子的例子)
3 Prox Calculus Rules(临近算子的计算规则)
可分函数:(x又是向量)
f 的缩放与平移(仅要求proper)
f proper,closed,convex
4 Prox of Indicators—Orthogonal Projections(指示函数的临近算子-正交投影)
4.1 The First Projection Theorem
正交投影算子:
The first projection theorem:the orthogonal projection mapping (which coincides with the proximal mapping)exists and is unique.
正交投影映射与邻近映射一致
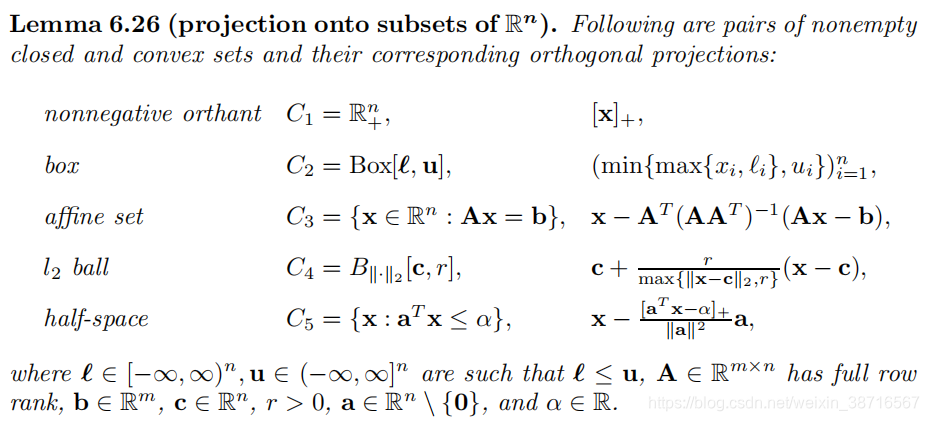
4.2 First Examples in 
4.3 Projection onto the Intersection of a Hyperplane and a Box
4.4 Projection onto Level Sets
4.5 Projection onto Epigraphs
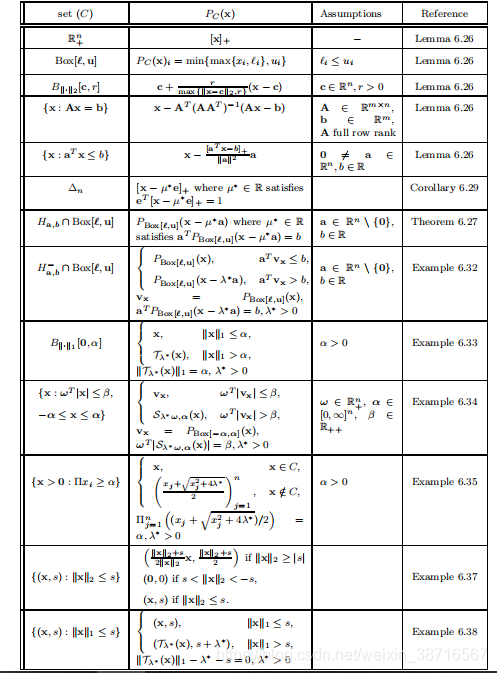
4.6 总结
5 The Second Prox Theorem
证明利用到如下Fermat最优化条件
第二投影定理:
临近梯度算子的稳定非扩张性:
6 Moreau Decomposition
闭凸函数中的prox算子与其共轭联系起来
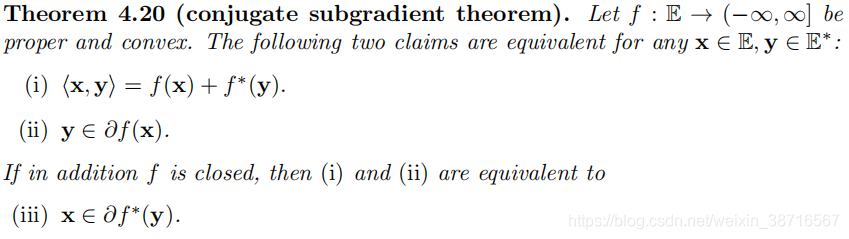
由如下共轭次梯度定理推导
增广的Moreau分解:
6.1 Support Functions
7 The Moreau Envelope(Moreau包络)
7.1 Definition and Basic Properties
μ is called the smoothing parameter.
上式由于第一临近定理,是有唯一解的,可得如下结论:
7.2 Differentiability of the Moreau Envelope
7.3 Prox of the Moreau Envelope
8 Miscellaneous Prox Computations