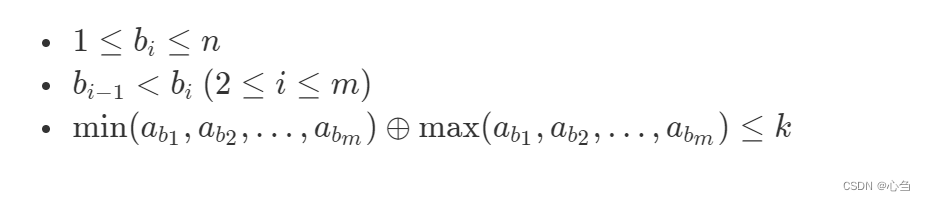题目
Tokitsukaze 有一个长度为 n 的序列 a1,a2,…,an和一个整数 k。
她想知道有多少种序列 b1,b2,…,bm满足:
其中 ⊕\oplus⊕ 为按位异或,具体参见 百度百科:异或
答案可能很大,请输出 mod1e9+7 后的结果。
输入描述:
第一行包含一个整数 T(1≤T≤2e5),表示 T 组测试数据。 对于每组测试数据: 第一行包含两个整数 n, k (1≤n≤2⋅e5; 0≤k≤1e9)。 第二行包含 nnn 个整数 a1,a2,…,an (0≤ai≤1e9)。
输出描述:
对于每组测试数据,输出一个整数,表示答案 mod1e9+7 后的结果。
输入
3 3 2 1 3 2 5 3 1 3 5 2 4 5 0 0 0 0 0 0
输出
6 10 31
思路
容易知道 b1,…,bm 实际上是 a 的一个子序列,并且由于我们只关注子序列中的最大值和最小值,因此可以先对 a 从小到大排序,再选择子序列。接着对子序列中的最大值进行分类,可以分成 n 类。即从左到右依次枚举 ai 作为子序列中的最大值,那么最小值就会在 aj, j∈[0,i] 中选。当满足 ai⊕aj≤k,那么以 ai 为最大值,aj 为最小值的子序列的数量就是 2的max{0,i−j−1}次方,特别的当 i=j 时答案为 1。
暴力的做法就是逐个枚举 aj 判断是否满足条件,时间复杂度是 O(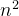
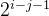
,因此在把 aj 按位插入 trie 中时,同时在对应节点加上
。
枚举到 ai 时,此时已经往 trie 中插入了 a0∼ai−1,枚举 ai 的每一位,用 xi 和 ki 分别表示 ai 和 k 在二进制下第 i 位上的值,s表示累加和。
1.当xi=1,ki=1时,显然另一数aj这一位是1的情况都是可以的,因为 1⊕1=0<1,所以s加上这一位为1的节点的值了,下一步走0节点。
2.当xi=0,ki=1时,同理s加上0节点的值,下一步走1节点.
3.当xi=1,ki=0时,0节点必然不成立,因为 1⊕0=1>0,下一步走1节点。
4.当xi=0,ki=0时,同理,1节点必然不成立,下一步走0节点。
最后以 ai 为最大值的子序列的数量就是。
代码
#include<bits/stdc++.h>
using namespace std;
#define int long long
//#define double long double
typedef long long ll;
const int N = 2e5+100;
const int mod = 1e9+7;
const int INF = 0x3f3f3f3f3f3f3f;
//ios::sync_with_stdio(0); cin.tie(0); cout.tie(0);
int a[N];
int to[N * 35][2];
int val[N * 35];
int tot = 0;
void insert(int x,int c) {
int p = 0;
for (int i = 30; i >= 0; i--) {
int v = (x >> i & 1);
if (!to[p][v]) {
to[p][v] = ++tot;
}
val[to[p][v]] = (val[to[p][v]] + c) % mod;
p = to[p][v];
}
}
int sum(int x,int k) {
int p = 0, res = 0;
for (int i = 30; i >= 0; i--) {
int vx = (x >> i & 1), vk = (k >> i & 1);
if (vk == 1 && vx == 1) {
res = (res + val[to[p][1]]) % mod;
p = to[p][0];
} else if (vk == 1 && vx == 0) {
res = (res + val[to[p][0]]) % mod;
p = to[p][1];
} else {
p = to[p][vx];
}
if (!p) break;
if (!i) res = (res + val[p]) % mod;
}
return res;
}
int ksm(int x,int n) {
int res = 1;
while (n) {
if (n & 1) res = res * x % mod;
x = x * x % mod;
n >>= 1;
}
return res;
}
void solve() {
int n,k;
cin >> n >> k;
for(int i=0;i<n;i++)cin>>a[i];
sort(a,a+n);
tot = 0;
for (int i = 0; i <= n * 32; i++) {
val[i] = 0;
to[i][0] = to[i][1] = 0;
}
int ans = 0;
for (int i = 1; i <= n; i++) {
ans = (ans + 1 + ksm(2,i - 1) * sum(a[i - 1],k) % mod) % mod;
insert(a[i - 1],ksm(ksm(2,i),mod - 2));
}
cout << ans << '\n';
}
signed main() {
ios::sync_with_stdio(0);
cin.tie(0);
cout.tie(0);
int t=1;
cin>>t;
while(t--)solve();
return 0;
}