Leetcode2427问题描述：

给你两个正整数 a 和 b ，返回 a 和 b 的 公 因子的数目。

如果 x 可以同时整除 a 和 b ，则认为 x 是 a 和 b 的一个 公因子 。

示例 1：

输入：a = 12, b = 6
输出：4
解释：12 和 6 的公因子是 1、2、3、6 。

示例 2：

输入：a = 25, b = 30
输出：2
解释：25 和 30 的公因子是 1、5 。

提示：

• 1 <= a, b <= 1000

Leetcode.728 问题描述

自除数 是指可以被它包含的每一位数整除的数。

• 例如，128 是一个 自除数 ，因为 128 % 1 == 0，128 % 2 == 0，128 % 8 == 0。

自除数 不允许包含 0 。

给定两个整数 left 和 right ，返回一个列表，列表的元素是范围 [left, right] 内所有的 自除数 。

示例 1：

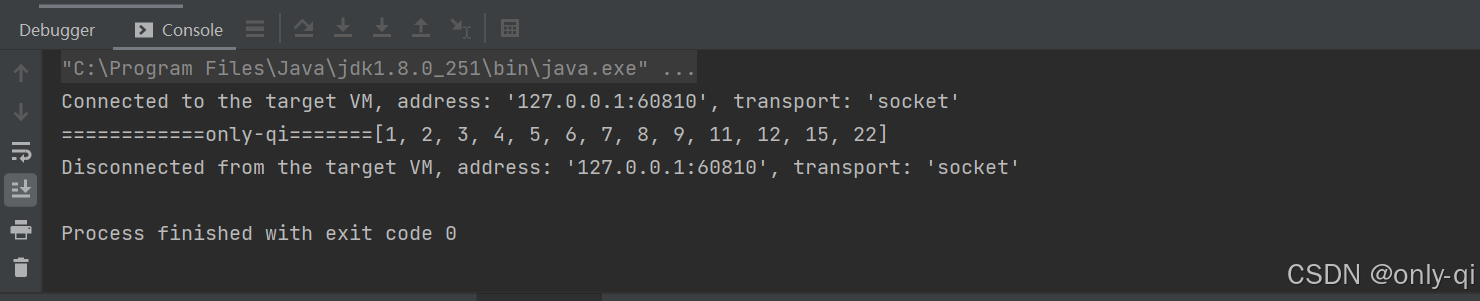
输入：left = 1, right = 22
输出：[1, 2, 3, 4, 5, 6, 7, 8, 9, 11, 12, 15, 22]

示例 2:

输入：left = 47, right = 85
输出：[48,55,66,77]

提示：

• 1 <= left <= right <= 104

代码拿去即可运行：

package com.onlyqi.daydayupgo01.test;

import java.util.ArrayList;
import java.util.List;

public class Test12 {
    public static void main(String[] args) {
        System.out.println("============only-qi======="+selfDividingNumbers(1,22));
    }
    public static List<Integer> selfDividingNumbers(int left, int right) {
        List<Integer> list=new ArrayList<>();
        for (int i = left; i <= right; i++) {
            int numTem=i;
            while (numTem>0){
                int temp=numTem%10;
                if(temp!=0 && i%(temp)==0){
                    numTem=numTem/10;
                    if(numTem==0){
                        list.add(i);
                    }
                }else {
                    numTem=0;
                }
            }

        }
        return list;

    }
    public static int commonFactors(int a, int b) {
        int count=0;
        for(int i=1;i<=Math.min(a,b);i++){
            if(a%i==0&&b%i==0){
                count++;
            }
        }
        return count;
    }

    public static int smallNums(int num) {
        int count=0;
        while(num>1){
            count=count+num/2;
            if(num%2==0){
                num=num/2;
            }else {
                num=num/2+1;
            }
        }
        return count;
    }
}

运行结果：

我要刷300道算法题，第117道 。 好久好久没写算法了，最近开始写，先从最简单的开始。希望自己可以坚持下去。