代码随想录算法训练营第十二天| 二叉树层序遍历 226.翻转二叉树 101. 对称二叉树

层序遍历

只使用二叉树无法完成层序遍历，需要借助其他数据结构，使用的是队列。首先，插入根结点，然后设置循环条件（一般来说，需要借助队列/堆栈实现的循环，循环条件都会包含这些数据结构）。记录遍历完每层后结点的数量（可以设置size为容器大小值，表示当前层结点的数量），size用于防止队列上本层结点与下一层结点混肴。

102. 二叉树的层序遍历

出现错误：
1.直接使用if(!root)而不是if（root！=NULL）

2.对于队列来说，获取队列头元素是front函数，而不是top

class Solution {
public:
    vector<vector<int>> levelOrder(TreeNode* root) {
        queue<TreeNode*> que;
        vector<vector<int>> res;
        if(root!=NULL) que.push(root);
        while(!que.empty()){
            vector<int> vec;
            int size=que.size();
            while(size--){
                TreeNode* node=que.front();
                que.pop();
                vec.push_back(node->val);
                if(node->left)que.push(node->left);
                if(node->right)que.push(node->right);
            }
            res.push_back(vec);
        }
        return res;
    }
};

107. 二叉树的层序遍历 II

对比前面只需要添加一个翻转函数

class Solution {
public:
    vector<vector<int>> levelOrderBottom(TreeNode* root) {
        queue<TreeNode*> que;
        vector<vector<int>> res;
        if(root!=NULL) que.push(root);
        while(!que.empty()){
            int size=que.size();
            vector<int> vec;
            while(size--){
                TreeNode* node=que.front();
                que.pop();
                vec.push_back(node->val);
                if(node->left) que.push(node->left);
                if(node->right) que.push(node->right);
            }
            res.push_back(vec);
        }
        reverse(res.begin(),res.end());
        return res;
    }
};

226. 翻转二叉树

层序遍历法

本题之前使用层序遍历的方式，还是使用size记录当前层的结点数量，然后当队列加入下一层子结点后，将队列弹出的node的左右子节点交换，用来实现结点交换

class Solution {
public:
    TreeNode* invertTree(TreeNode* root) {
        queue<TreeNode*> que;
        if(root)que.push(root);
        while(!que.empty()){
            int size=que.size();
            for(int i=0;i<size;i++){
                TreeNode* node=que.front();
                TreeNode* tmp;
                que.pop();
                if(node->right)que.push(node->right);
                if(node->left)que.push(node->left);
                tmp=node->left;
                node->left=node->right;
                node->right=tmp;
            }
        }
        return root;
    }
};

递归法

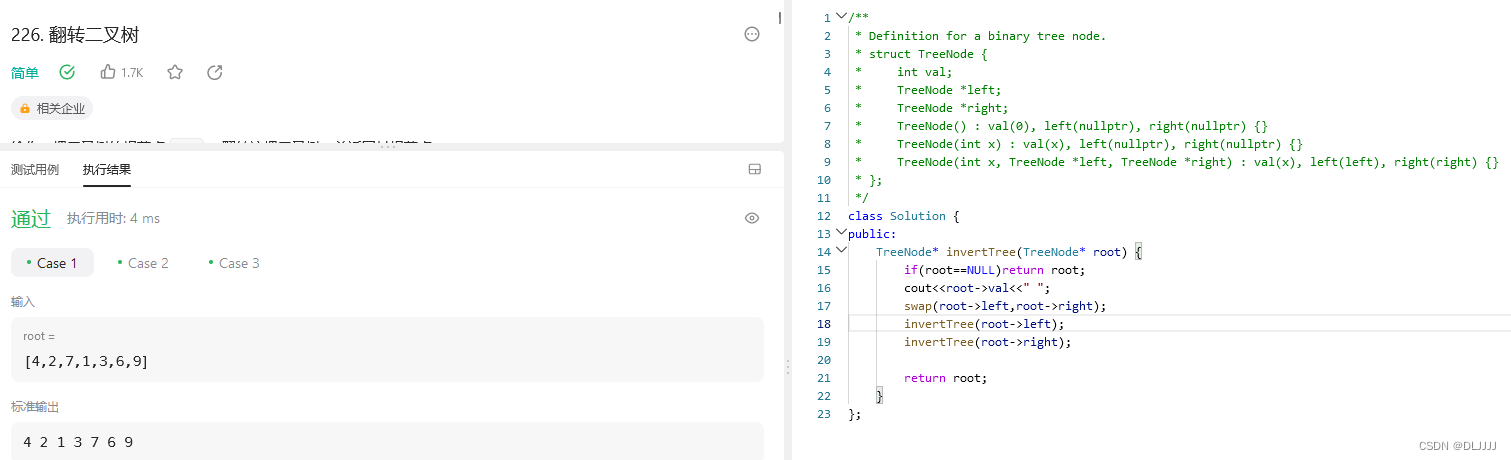
还有一种使用递归的方法进行翻转，需要掌握递归的方法，首先确定递归函数的参数和返回值，由题目可得参数类型以及返回值，然后确定循环终止条件，当此时结点为空时跳出当前层，然后再根据递归逻辑确立。本题可以使用前序遍历和后序遍历，直接使用中序遍历时会出错，因为中序遍历会照成结点翻转两次。

在本题中，通过打印结果可以看到递归遍历的结点，首先将左右对换后，再遍历对换后的左结点，然后再右结点所以输出出来结果是反着的。

class Solution {
public:
    TreeNode* invertTree(TreeNode* root) {
        if(root==NULL)return root;
        cout<<root->val<<" ";
        swap(root->left,root->right);
        invertTree(root->left);
        invertTree(root->right);

        return root;
    }
};

101. 对称二叉树

层序遍历法

使用层序遍历可以完成，在层序遍历中，第一次不需要将头结点放入队列中，而是可以将其子节点放入队列中，然后根据队列上面左右节点判断其是否存在，是否相同，最后再将子节点中的子结点按照对称的顺序放入队列中即可。

class Solution {
public:
    bool isSymmetric(TreeNode* root) {
        queue<TreeNode*> que;
        if(root==NULL)return true;
        que.push(root->left);   // 将左子树头结点加入队列
        que.push(root->right);  // 将右子树头结点加入队列
        while(!que.empty()){
            TreeNode* left=que.front();
            que.pop();
            TreeNode* right=que.front();
            que.pop();
            if(!left&&!right)continue;
            if ((!left || !right || (left->val != right->val))) {
                return false;//要分析好left和right的情况，因为不可能同时为0，所以只要有一个为0，就会返回false
            }
            que.push(left->left);
            que.push(right->right);
            que.push(left->right);
            que.push(right->left);
        }
        return true;
    }
};

递归法

确定递归顺序。本题使用后序，使用后序遍历的情况：收集孩子信息向上一结点返回。

1.首先确定函数参数类型以及返回值：因为要比较的是根节点的两个子树是否是相互翻转的，进而判断这个树是不是对称树（因此确定函数参数类型为bool），所以要比较的是两个树，参数自然也是左子树节点和右子树节点。

2.判断终止条件：

左节点为空，右节点不为空，不对称，return false
左不为空，右为空，不对称 return false
左右都为空，对称，返回true
左右不为空，但是值不同，return false

剩下的就是左右节点都不为空，且数值相同的情况，然后以此进行判断

3.确定单层递归的逻辑

比较二叉树外侧是否对称：传入的是左节点的左孩子，右节点的右孩子。
比较内侧是否对称，传入左节点的右孩子，右节点的左孩子。
如果左右都对称就返回true ，有一侧不对称就返回false 。

class Solution {
public:
    bool compare(TreeNode* left,TreeNode* right){
        if(!left&&right)return false;
        else if(left&&!right)return false;
        else if(!left&&!right)return true;
        else if(left->val!=right->val)return false;
        bool outside=compare(left->left,right->right);//左
        bool inside=compare(left->right,right->left);//右
        return outside&&inside;//中
    }
    bool isSymmetric(TreeNode* root) {
        if(!root)return true;
        return compare(root->left,root->right);
    }
};